Pervasive sensing provides a new paradigm for monitoring, modeling and control of natural and infrastructure systems that affect the environment. CENSAM aims to create a center of excellence in environmental sensing and modeling that will demonstrate the importance of pervasive sensing through applications in the well managed urban environment in Singapore.

Click here for more information about this project.

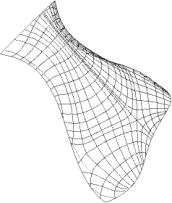
The objective of this project is to develop an intrinsic watermark technique for solids bounded by NURBS surfaces. The key idea is to extract intrinsic properties of solids, which are not affected by coordinate transformations, random noise and malicious action of the user. This watermark can be destroyed only if the digital model describing the shape is changed so much that the newly represented object cannot any longer be considered approximately identical to the original solid in the database.

Click here for more information about this project.

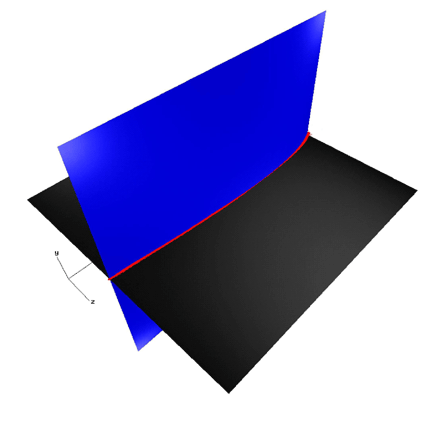
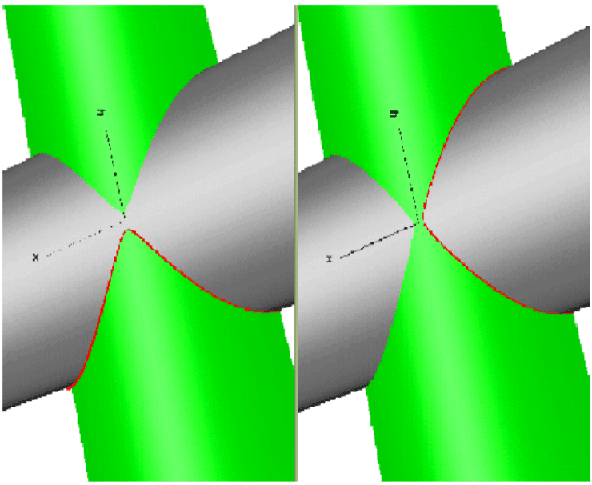
Full automation of the design of complex structures requires reliable geometric computations. This reliability is absent from current CAD/CAM systems due to their implementation in floating point arithmetic, which leads to inconsistent and unstable numerical computations. We are developing a solid modeling system based on interval splines, which differ from classical splines in that the control point coordinates are represented as intervals, which in combination with rounded interval arithmetic guarantees the robustness of geometric computations. A current project involves conversion of legacy geometric models to robust interval solid models that are topologically valid and geometrically consistent. Producing rectified models free from gaps and inappropriate intersections is necessary for effective analysis and manufacturing.

Click here for more information about I-TANGO.
Click here for more information about SGER.

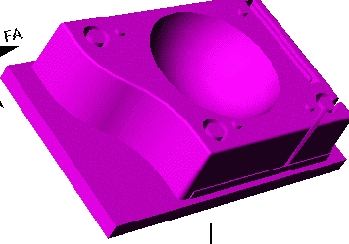
With the capability for manufacturing parts with variable material composition, Solid Freeform Fabrication (SFF) processes are moving beyond the capabilities of state-of-the-art solid modelers. For designers to have immediate access to this new technology, methods need to be developed to facilitate accurate representation, design, and processing of parts with graded material composition. The four major areas of this research include the development of a solid modeling method incorporating graded composition information, composition design tools, processing algorithms to drive the manufacturing process, and design rules incorporating process limitations that will be used to evaluate models for manufacturability.

Click here for more information about 3D Printing.
Click here for 3DP Laboratory.

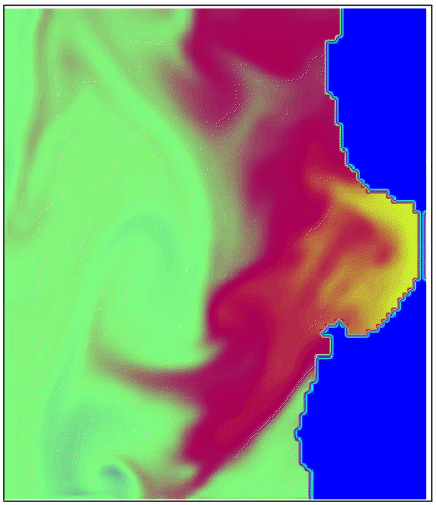
Click here to link to the Poseidon project page.

Click here to link to the Laser Line Heating Page (Fabrication Laboratory).

Inspection is the process of verifying shape conformance of a measured manufactured part with a toleranced geometric description of a design object. Since milling and casting processes are inaccurate, the need for accurate inspection methods is fundamental, particularly for objects that require a very high level of precision. Inspection is performed by feature extraction, which extracts performance related geometric features from a mathematical design or as--built model; localization, which optimally positions a mathematical design model with respect to a measured manufactured artifact; and local error analysis, which is used for planning remachining operations. A current project involves shape reconstruction of curved objects using interval B-splines.

In order that the design and manufacturing processes be interactive, algorithms developed in the MIT Design Laboratory for shape creation, advanced interrogation, visualization, fairing, manufacturing, and inspection have been integrated into an executive system called Praxiteles. Praxiteles provides visualization facilities for numerical computations, and input and output via the IGES format, the standard for geometric data exchange. Praxiteles facilitates the exchange of curve and surface geometry between modeling systems through advanced methods for approximate conversions of high degree Bezier and B-spline surfaces to lower degree representations with guaranteed bounds on the approximating error.

Click here for more information about Praxiteles.

Developable surfaces can be manufactured by bending flat plates without stretching or tearing, i.e. by rolling. Their use for ship construction has the advantages of lower labor costs, smaller capital investment, ease of repair and simple tools for construction. We have investigated their design and representation by NURBS surfaces and how to accurately develop them onto a plane. Future projects include the automatic derivation of roller instructions for the fabrication of plates and issues of accuracy control.

We have developed a statistical model for evaluating the relative risk of ship transit through the nation's ports and waterways. The model is expected to lead to rational criteria for estimating hydrographic survey priorities. It predicts the relative risk of physical accidents and, more importantly, the economic risk of such occurrences in terms of cargo loss and environmental damage. Projects include the effects of waterway changes (geometry, aids to navigation, introduction of port management systems, etc.) on ship transit risk.

This project developed piecewise linear approximation methods for the edges and faces of Boundary Representation (B-Rep) solid models. Our method is based on robust geometric definitions and computations, and the existence of a homeomorphism between the exact geometry and its approximation. The approximation consists of an unstructured adaptive triangular mesh. This research has important application for finite element meshing, data exchange, visualization, SFF, and geometric computations.

A fundamental problem in computer aided design is the robust and efficient computation of all real roots of systems of nonlinear polynomial equations. Such problems often occur in computing intersections, distance functions and extrema, and in engineering design. In order to isolate all roots within a given domain our method projects control polyhedra onto a set of coordinate planes and uses rounded interval arithmetic for numerical robustness. Current projects include computing self--intersections of offset curves and surfaces, useful for NC machining, and the rational polynomial representation of pipe surfaces.

This project generates coarse and fine meshes on multiply connected 2D and 3D domains. We have studied the MAT (medial axis transform), which is a point set consisting of points equidistant from two or more points on the boundary contour. Using the MAT, we can extract important shape characteristics and length scales that are used to create a coarse subdivision of a complex surface. Additionally, we can generate fine meshes within individual subregions. This can lead to integration of automated finite element (FE) mesh generation schemes into CAD systems.